The ratio to the class would mean the following: How many men are there in comparison to the class as a whole (men and women combined).
Breaking down the word problem, you're essentially left with two operations: division and multiplication.
Answer 2 is the only answer in which the two ratios would be the same with a common denominator.
The equation for determining slope is as follows: m=y2 - y1x2 - x1 where x1 is not equal to x2.
The equation for determining slope is as follows: m=y2 - y1/x2 - x1 where x1 is not equal to x2.
Use the equation a2 + b2 = c2, plug in the known values, and solve.
Supplementary angles must add up to 180 degrees. Using that logic, you can solve for x.
Advertisement
The following can help you determine midpoint coordinates: x1+x2/2, y1+y2/2
To determine the area of a triangle, use the equation A=1/2bh (or base times height) The problem gives you the area as being 110 square inches. Plug that in for A. Next, plug in 15 inches for base (also given) and solve for h.
The total amount of costs will be represented by the price quote, P. Given that a base charge of $25 is assumed, we may start by stating the price quote as P = $25. Our price quote is now P = $25 + $8B, which adds $8 for each bathroom, denoted by the variable B, to the $25 base payment. The next step is to add $4 for every additional room or R, bringing the total price to P = $25 + $8B + $4R.
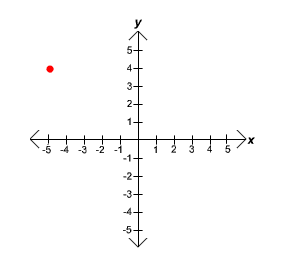
The origin, denoted as (0, 0), where the horizontal x-axis and vertical y-axis intersect, forms the center of the coordinate plane. The coordinate system (x, y) is used to represent points on a plane that have both an x coordinate and a y coordinate. Positive and negative x values are above and below the origin, respectively, whereas negative and positive y values are to the left and right of the origin. In this instance, the point lies above and to the left of the origin. The only response option with a negative x coordinate must be the right one.
This is a function issue, where a function connects an input to an output. To find (2.5), replace every x in the original function with 2.5, then evaluate the following expression:
ƒ(2.5) = 2(2.5)2 − 3(2.5) +7
ƒ(2.5) = 2(6.25) − 7.5 + 7
ƒ(2.5) = 12.5 − 7.5 + 7
ƒ(2.5) = 12
After simplifying the expressions using the PEMDAS method, combine like terms before isolating the variable. Equations and inequality can be treated similarly, but there is one key distinction: when multiplying or dividing either side of an inequality by a negative value, the inequality symbol's direction must change.
In this instance, multiply the number three via the parenthesis first before separating the variable:
5x + 8 < 3(x + 2)
5x + 8 < 3x + 6
2x + 8 < 6
2x < −2
x < −1
Recall that the multiplication of terms sharing the same base can be simplified by adding the exponents: x^a * x^b = x^(a + b). If you forget this rule, you can easily derive it by thinking about the straightforward instance of 2^1 * 2^2, which can also be represented as 2 * 2 * 2 or 2^3. Therefore, the phrase in this instance can be summarised as follows:
(x^6) (x^5) = x^5+6
= x^11
Advertisement
Area = π * r^2
where "r" is the radius of the circle.
In the given information, the area of the circle is 100π. So we have:
100π = π * r^2
Now, let's solve for ""r"":
r^2 = 100
Taking the square root of both sides:
r = √100
r = 10
Now, we know the radius (r) of the circle is 10 units. And the diameter (D) is twice the radius:
D = 2 * r
D = 2 * 10
D = 20
Average = total data points divided by total data points
75 = (50 + 52 + 77 + 88 + 91 + x) ÷ 6
Now calculate x. Begin by adding:
75 = (358 + x) ÷ 6
Next, multiply both sides by 6 to remove the denominator:
450 = 358 + x
The final stage is to take 358 away from each side.
x = 92
The absolute value of the difference is indicated by the vertical bars surrounding a and b. The distance between a simplified expression and 0 is an expression's absolute value, which is always positive. In the expression, replace the given numbers for a and b, calculate the difference, and then add the absolute value to make the expression positive.
|a − b|
= |−4 − 3|
= |−7| = 7
Remember that −4 − 3 is equivalent to −4 + −3 = −7
Number of men = 4
Total number of individuals = 4 (men) + 8 (women) = 12
Now, the probability of choosing a man is given by:
Probability = (Number of men) / (Total number of individuals)
Probability = 4 / 12
Now, let's simplify the fraction:
Probability = 1/3
If 46% of the total number of students is female, then 100% − 46% = 54% of the students are male. To determine the proportion of male pupils, divide the total by 54 percent.
Recall that 54% is equivalent to 54 ÷ 100, or 0.54:
0.54 * 1250 = 675.
Calculate the two months with the biggest difference by deducting each expense in the spending column from its corresponding revenue.
January: $3020 − $2370 = $650
February: $2827 − $2012 = $815
March: $3589 − $2620 = $969
April: $3424 − $2630 = $794
May: $3540 − $2570 = $970
Between March and May, Erin saves the most money.
Click for the next FREE GED Test