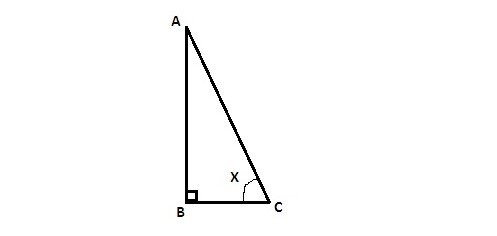
In the given triangle, we are given the tangent of an angle as tan(x)=40/9, which represents the ratio of the side opposite the angle (BC) to the side adjacent to the angle (AB). Using the Pythagorean theorem with the known values of AB and BC, we find that the length of side AC is 41 units.
A positive angle is measured in a counterclockwise direction from the starting point to the ending point of the angle. Starting from the initial side, you rotate in the counterclockwise direction to reach the terminal side, and the measurement of this rotation gives the measure of the positive angle.
One revolution in degrees is equal to 360 degrees. In other words, if you make a complete circle, you have completed one full revolution, and the total angle covered is 360 degrees.
Degrees are widely used to measure angles in various fields, including mathematics, physics, engineering, and everyday life. However, there is another unit called radians that is also commonly used, especially in advanced mathematics and physics. Radians are particularly useful when dealing with trigonometric functions and calculus, as they simplify many mathematical expressions involving angles. But in general, degrees remain the more familiar and commonly used unit for measuring angles in most contexts.
The value of π (pi) is approximately 3.14159. It is an irrational number, which means it cannot be expressed as a simple fraction, and its decimal representation goes on infinitely without repeating. In many mathematical calculations and formulas, π is commonly approximated to 3.14 for simplicity, but its precise value is 3.14159 and continues indefinitely.
One revolution corresponds to a complete circle, and in terms of radians, it is equal to 2π radians. This is because the circumference of a circle is 2π times its radius, and since a complete circle is formed by going around the entire circumference, it covers 2π radians. So, the measure of an angle in radians for one revolution (a full circle) is 2π radians
In a right triangle with side lengths a=8 and b=6, the Pythagorean theorem (a^2+b^2=c^2) is used to find the length of the hypotenuse (c). After calculating c=10, the cosine of angle α (cos(α)) is determined by dividing the side adjacent to the angle (b) by the hypotenuse (c), resulting in cos(α)=3/5.
Advertisement
In a right triangle with side lengths a=3 and c=5, we use the Pythagorean theorem (a^2+b^2=c^2) to find the length of the remaining side (b). After calculating b=4, we can determine the tangent of angle α (tan(α)) by dividing the side opposite the angle (a) by the adjacent side (b), resulting in tan(α)=3/4.
To convert degrees to radians, we use the conversion factor π radians = 180 degrees.
Given 360°, we can use the conversion factor as follows:
360° = 360/180 x π radians 2π radians.
So, 360° is equivalent to 2π radians.
To find the length of the hypotenuse (c) in the right triangle with side lengths =10 and b=12, we use the Pythagorean theorem, which states that the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the two legs. Plugging in the given values, we get c^2=10^2+12^2. Simplifying further, c^2=100+144, and thus c^2=244. Now, to find the actual length of the hypotenuse (c), we take the square root of both sides: c=√244. Further simplifying, we find c=√4×61. Using the property √ab=√a×√b, we get c=√4×√61. Finally, simplifying further, we arrive at the correct length of the hypotenuse (c), which is 2√61.
Imagine you are standing on the ground, and there's a tree in front of you. You are 47 feet away from the base of the tree. If you look up, the angle formed between your line of sight and the ground is 35 degrees. Now, we want to find out how tall the tree is. To do that, we use trigonometry, which is like using special math rules for triangles. The tangent function allows us to relate the height of the tree to the distance you are from the tree and the angle you're looking up. By plugging the values into the tangent equation, we find that the height of the tree is approximately 32.91 feet when rounded to the nearest foot. So, the tree's height is about 33 feet.
In this scenario, you have a right triangle where the distance from you to the base of the platform is the horizontal side, the height of the platform is the vertical side (denoted as "h"), and the line of sight from you to the top of the platform is the hypotenuse. By using trigonometry, specifically the tangent function, we can find the height of the platform ("h") based on the given angle of elevation (62 degrees) and the distance from the base (200 feet).
Using the tangent function, we have:
Tan (62°) = height/distance = h/200.
Now, we can solve for "h" by multiplying both sides by 200:
ℎ=200 × tan (62°).
Using a calculator, we find:
ℎ≈200 × 1.880726 ≈ 376.15
The height of the platform is approximately 376.15 feet when rounded to the nearest foot.
To find the height of the flagpole (h), given the shadow length of 10 feet and an angle of elevation of 32 degrees, we use the tangent function in a right triangle formed by the flagpole, its shadow, and the line from the top of the flagpole to the sun. The tangent function relates the height (opposite side) to the shadow length (adjacent side) using the angle of elevation.
Using the equation h ≈ 10 x tan (32°)
and using a calculator , we find:
H ≈ 10 x 0.6249 ≈ 6.249.
Make sure to round to 2 places after the decimal.
The height of the flagpole is approximately 6.25 feet when rounded to the nearest foot.
To find out how far up the ladder goes, we use the sine function:
Sin (70°) = height/125,
where "height" represents the distance up the side of the house.
To find the height, we can rearrange the equation:
height = 125 × sin (70°).
Using a calculator, we get:
Height ≈ 125 × 0.9397 ≈ 117.46.
So, the height that the ladder reaches is approximately 117.46 feet when rounded to two decimal places.
Advertisement
In right triangle ABC, where angle A measures 90 degrees, we need to find the length of side BC. To do this, we can use the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Given that side AB measures 15 units and side AC measures 36 units, we can set up the equation:
AB^2+AC^2=BC^2.
Substituting the given values, we get:
15^2+36^2=BC^2.
Solving the equation:
225+1296=BC^2,
we find:
BC^2=1521.
Taking the square root of both sides to find the length of side BC:
BC = √1521 = 39.
So, the length of side BC in triangle ABC is 39 units (feet, inches, etc.).
To find the length of the zip wire (H), we can use the formula:
H= A / cos(θ),
where A is the distance between the two posts (25 meters), and θ is the angle between the zip wire and the horizontal (10 degrees).
Substitute the given values:
H= 25 / cos (10)
Using a calculator, we find:
H= 25/0.9848 = 25.4.
So, the length of the zip wire is approximately 25.4 meters when rounded to one decimal place.