Explanation:
This question assesses your capacity to comprehend the idea and calculate percentages to tackle problems. In order to respond to this query, we must first figure out how much Henry spent for each item separately and then combine those figures. The toaster cost $100, but after the 15% reduction, it will cost you only $85. In addition, the $1200 oven gets a 15% reduction first: (1200 x 85) / 100 = $1020. But this item also qualifies for an additional 25% member discount: (1020 x 75) / 100 = $765. By adding the two prices, we can determine that Henry spent $850 for both things (i.e. $85 + $765).
Explanation:
The purpose of this kind of question is to see how well you comprehend the idea of successive numbers. Ascending whole numbers are consecutive numbers, like 1, 2, 3, and 4. If the first of the four numbers is denoted by the symbol X, then the second number is denoted by X + 1, the third by X + 2, and the fourth by X + 3. If all four numbers added together add up to 18, then X + (X + 1) + (X + 2) + (X + 3) = 18. Consequently, 4X = 18 - 6 = 12 or 4X = 1 + 2 + 3 = 18. 4X Equals 12, thus X must equal 3. As a result, our lowest four consecutive number must be 3, and the highest must be 6.
Explanation:
To answer this question, we must first eliminate the five people who do not enjoy sports at all: 35 - 5 = 30. Based on the facts in the question, we can infer that 17 staff employees enjoy playing rugby, and 22 enjoy playing basketball, for a total of 39—9 more than the number of people we know enjoy playing at least one sport. This implies that those 9 must enjoy both basketball and rugby.
Explanation:
Converting the data into two simple equations is the quickest way to find the answer to this problem. This quiz tests your ability to conceptualize numerical data as equations. So let's change Amy to A and Shelly to S. Now that we have the second clause, we can construct the equation shown below: S = 2A + 10 can be written as A + 5 = S / 2. The following sentence allows us to create another equation: S = 4A - 20 is the result of simplifying A - 5 = S / 4. These equations can now be easily expressed in terms of A: 4A - 20 = 2A + 10, which equals S. All of the numbers can now be placed on one side of the equation, and all of the variables on the other: 4A - 2A = 20 + 10 Consequently, 2A = 30 and A (Amy) = 15.
Explanation:
This question is designed to test your comprehension of whole numbers. Whole numbers can be positive or negative and lack the decimal point. We must take into account the fact that the least whole number of cards that each player can possess is 1, in order to determine the minimal number of cards that our four players can possess. As a result, each player can only have a maximum of 6 x 1, 3 x 1, 5 x 1, and 4 x 1 cards in their turn. This means that each player must hold a minimum of six, three, five, and four cards, for a total of 18. Although it is certainly conceivable for them to each have more cards based on greater whole numbers, the question merely specifies the "minimum" number that is possible.
Explanation:
This is a well-known question that may frequently be resolved intuitively or by applying a few very basic equations. Based on the first half of the first statement, we may create the following equation if we use equations: Tom = 2 times Steve We may create a second equation from the second half of the sentence: Nat x 4 = Steve Using the first equation, if Tom = 40, we can deduce that Steve = Tom / 2 = 40 / 2 = 20. Nat = Steve / 4 = 20 / 4 = 5, which we can now swap for the number we discovered for Steve to determine Nat's sales.
Explanation:
Your capacity to employ simple mathematical calculations to find solutions is evaluated by this question. You can answer this question by visualizing the fence as a 48-meter straight line (i.e. 12m x 4 sides). We can easily determine the necessary number of posts by dividing the overall length by the requisite gap size, or 48 / 3 = 16, since there must be a 3m space between neighboring posts. It is crucial to keep in mind that in order to hold the final section of the fence in place, we would require one more post. The last post is not necessary because the first post also supports the last portion of the fence because the farmer has the fence enclosed.
Advertisement
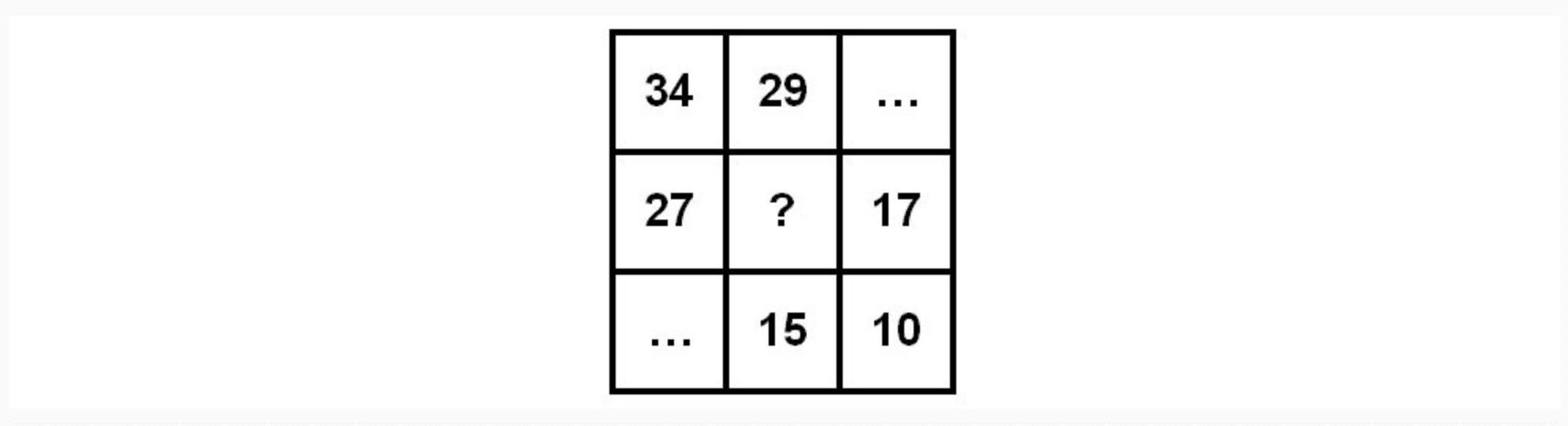
Explanation:
This question is designed to see how well you can spot patterns in a collection of numbers. The number groups become number series as a result of these patterns. Three rows and three columns of numbers make up this table. The number series that each row and each column represent are the same, yet the series in the rows and columns may not be the same. The top line reveals that there is a 5 discrepancy between the two values (i.e. 34 – 29). Likewise, the difference between the two values in the final calculation is also 5. (15 – 10). This leads us to the conclusion that each number in these rows is decreased by 5 as we move from left to right. Applying that principle to the middle line, the? should equal 22. (i.e. 27 – 5). By subtracting 5 from 22, which results in 17, we can verify that the solution is correct. This matches to the last number given in the row.farmer has the fence enclosed.
Explanation:
This test question is designed to see how well you can spot patterns in a collection of numbers. The number groups become number series as a result of these patterns. Three rows and three columns of numbers make up this table. The number series that each row and each column represent are the same, yet the series in the rows and columns may not be the same. We then examine the variations between the adjacent integers in the middle and bottom rows in an effort to identify a pattern. This would result in a difference of 4 at the final step (24 - 20) in the middle row and a difference of 4 at the first step (10 - 6) in the bottom row, when viewed from left to right. Therefore, it appears that the trend is to add 4 at each stage, bringing the? value to 14 (i.e., 10 + 4). By completing the remaining empty squares, we can rapidly validate this. A different option would be to find the pattern in the columns. Going from top to bottom, the middle row shows a difference of 10 at the last step (20 - 10), whereas the last column shows a difference of 12 at the initial step. Finishing the next columns will allow us to test this: 28 16 6, 32 20 10, and 36 24 14. This further supports our choice of 14.
Explanation:
Find out how many chairs Gary can construct in 6 hours to get the solution to this question. Jill, Brian, and Gary can construct 240 chairs in 4.5 hours, according to the question. This suggests that they must be able to construct 480 chairs in total in 9 hours (we multiplied the two sides by 2 using the previous data: 240 chairs x 2 = 4.5 hours x 2). Gary must be able to manufacture the remaining 180 chairs because Jill and Brian will only be able to make 300 of those in the allotted 9 hours: 480 - 300 = 180 chairs. In other words, Gary will be working at a pace of 20 chairs per hour (180 chairs / 9 hours). Therefore, Gary will create 120 chairs in 6 hours (20 chairs x 6 hours).
Explanation:
This test question is designed to determine your level of comfort dealing with fractions and whole numbers, as well as your comprehension of what they mean. We must describe the fractions we have with the same basis in order to obtain minimal numbers because the minimum number for each breed must be a whole number. Therefore, 2/5 and 1/4 equal 8/20 and 5/20. (i.e. the common base is 5 x 4). This means that at least 5 of the minimum number of 20 dogs must be Golden Retrievers. Naturally, this also implies that there must be at least 7 more dogs present who are neither Cavaliers nor Golden Retrievers.