FREE MSAP Reasoning in the Sciences and Mathematics Questions and Answers

0%

Which one of the schedules meets the requirements of the Firefighters’ Union?

Correct! Wrong!

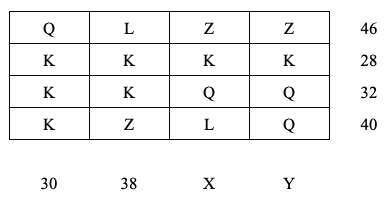
Explanation:
The schedules that meet the requirements of the Firefighters’ Union is column II.

The schedule {1,2}, {2,3}, {x,y} is a schedule for which v = 3, b = 3, k = 2, r = 2, if:

Correct! Wrong!

Explanation:
For the schedule to meet the conditions where each set contains two firefighters (k = 2) and each firefighter appears twice in the schedule (r = 2), the values of x and y must be chosen such that all firefighters are included twice. Since firefighters 1, 2, and 3 are involved, setting x = 1 and y = 3 ensures that all three firefighters are included twice, meeting the requirements.

What is the purpose of statistical analysis in social sciences research?

Correct! Wrong!

Explanation:
Statistical analysis in social sciences research is used to identify patterns, relationships, and trends in data. It helps researchers draw conclusions and make inferences about the population being studied.

In a scientific experiment, what does the control group allow researchers to do?

Correct! Wrong!

Explanation:
The control group provides a standard against which the experimental group, which receives the treatment or intervention, is compared. This helps researchers determine whether the results observed in the experimental group are due to the intervention or other factors.

In the grid below, the letters represent different whole numbers of less than 20. The numbers to the right and below the grid are row and column totals. The value of Q is:

Correct! Wrong!

Explanation:
The value of Q is 9.

If a car travels 200 kilometers in 4 hours, what is its average speed in kilometers per hour?

Correct! Wrong!

Explanation:
Average speed is calculated by dividing the total distance traveled by the total time taken.

A schedule can be thought of as a set of v objects (in this case, firefighters) that have to be arranged into b sets (in this case, one set for each day of the week) all of size k and such that each object occurs the same number of times (r) in the schedule and only once in any set. For the firefighters’ schedules, v = 7, b = 7, k = 3, and r = 3.

If v = 3, b = 6, k = 1, r = 2, which one of the following completes the schedule {1}, {2}, {3}, {1}, {2}, . . .?

Correct! Wrong!

Explanation:
Given that each set in the schedule should contain only one firefighter (as k = 1), and the requirement that each object occurs the same number of times in the schedule (r = 2), the firefighter that has not yet appeared in the schedule is 3. Therefore, adding Firefighter 3 to the next position completes the schedule correctly.

Which of the following is an example of qualitative data?

Correct! Wrong!

Explanation:
Qualitative data is descriptive and categorical rather than numerical. Color descriptions represent qualitative data, whereas options A, B, and D represent quantitative data.

What is the square root of 144?

Correct! Wrong!

Explanation:
The square root of 144 is 12, as 12 × 12 = 144.

Which mathematical concept is commonly used to measure the strength and direction of a relationship between two variables?

Correct! Wrong!

Explanation:
Correlation is a statistical measure that quantifies the strength and direction of the relationship between two variables. It ranges from -1 to +1, where +1 indicates a perfect positive correlation, -1 indicates a perfect negative correlation, and 0 indicates no correlation.

In a class of 25 students, 16 students are proficient in mathematics and 18 students are proficient in science. How many students are proficient in both mathematics and science?

Correct! Wrong!

Explanation:
This can be found by adding the number of students proficient in mathematics (16) and the number of students proficient in science (18), then subtracting the total number of students (25) to find the overlap.

Related Post