FREE HSA Mathematics Questions and Answers

0%

The level of the top of the water in the ocean is considered to be at an altitude of zero (0) feet.
‥ The ocean floor at a particular dive site is − 20 feet.
‥ A diver is located at − 5 feet at that same site.
‥ The captain of a boat is located at an altitude of 15 feet, directly above the diver.

When the diver swims to − 10 feet, the diver will be the same distance below the top of the water as the captain is above the top of the water.

Correct! Wrong!

Explanation:
When the diver swims to -10 feet, they are 10 feet below the top of the water (which is at 0 feet altitude). At the same time, the captain of the boat remains at an altitude of 15 feet above the top of the water. Therefore, the diver will not be at the same distance below the top of the water as the captain is above it.

Amanda asked a random sample of 40 students from her school to identify their birth month. There are 300 students in her school. Amanda’s data is shown in this table.

Which of these statements is best supported by the data?

Correct! Wrong!

Explanation:
Option C is correct, since 27.5% of students are born in April, and only 15% are born in July.

A carpenter used exactly 25 feet of wood to make 9 shelves of equal length. Each shelf measured between:

Correct! Wrong!

Explanation:
To find the length of each shelf, divide the total length of wood (25 feet) by the number of shelves (9).

25 feet/9 shelves ≈ 2.78 feet

So, each shelf measures approximately 2.78 feet.

Therefore, the correct answer is 2 and 3 feet.

The principal concluded that there was more variability in the number of books sold by Class 1 than Class 2. Which statement is true about the principal’s conclusion?

Correct! Wrong!

Explanation:
Statement 2 is true about the principal’s conclusion.

A company designed two rectangular maps of the same region. These maps are described below.

Map 1:
The dimensions are 8 inches by 10 inches.
The scale is 3/4 mile to 1 inch.

Map 2:
The dimensions are 4 inches by 5 inches.

Which ratio represents the scale on Map 2?

Correct! Wrong!

Explanation:
Let's find the scale for Map 2.

For Map 1, 1 inch = 3/4 mile.

Given that Map 2 has dimensions half of Map 1, its scale will be doubled.

So, for Map 2, 1 inch = 3/4 × 2 = 3/2 miles.

Therefore, the correct answer is: 3/4 mile to 1/2 inch.

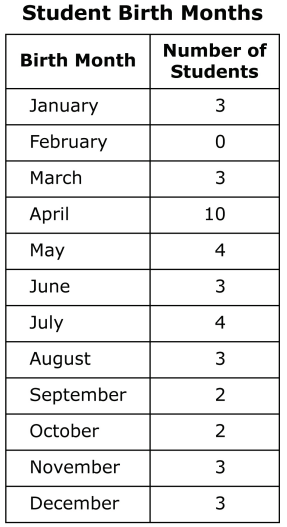
In a class of 30 students, 18 students scored above 80% in a math test. What percentage of the class scored above 80%?

Correct! Wrong!

Explanation:
To find the percentage of students who scored above 80%, divide the number of students who scored above 80% by the total number of students and multiply by 100: (18/30) * 100 = 60%.

In art class, Marvin painted tiles to use for a project. For every 5 tiles he painted blue, he painted 8 tiles green.

Identify the equivalent ratio(s) of blue tiles to green tiles.

Correct! Wrong!

Explanation:
60:96 is an equivalent ratio of blue tiles to green tiles.

If a rectangular swimming pool has a length of 20 meters and a width of 10 meters, what is the perimeter of the pool?

Correct! Wrong!

Explanation:
The perimeter of a rectangle is the sum of all its sides. For this pool, the perimeter would be 2 * (length + width) = 2 * (20 + 10) = 2 * 30 = 60 meters.

If the ratio of boys to girls in a class is 3:2 and there are 25 students in total, how many boys are there?

Correct! Wrong!

Explanation:
Divide the total number of students by the sum of the ratio parts (3 + 2) to find the value of each part. Then, multiply the number of parts representing boys (3) by this value: (3/5) * 25 = 15. So, there are 15 boys in the class.

The level of the top of the water in the ocean is considered to be at an altitude of zero (0) feet.
‥ The ocean floor at a particular dive site is − 20 feet.
‥ A diver is located at − 5 feet at that same site.
‥ The captain of a boat is located at an altitude of 15 feet, directly above the diver.

The distance from the captain to the diver is greater than the distance from the top of the water to the ocean floor.

Correct! Wrong!

Explanation:
The statement is False because the distance from the captain to the diver is the same as the distance from the top of the water to the ocean floor, both being 20 feet.

The level of the top of the water in the ocean is considered to be at an altitude of zero (0) feet.
‥ The ocean floor at a particular dive site is − 20 feet.
‥ A diver is located at − 5 feet at that same site.
‥ The captain of a boat is located at an altitude of 15 feet, directly above the diver.

The distance from the captain to the top of the water is the same as the distance from the diver to the ocean floor.

Correct! Wrong!

Explanation:
True. Since the diver is at -5 feet and the captain is at 15 feet, the vertical distance between them is 20 feet. This is the same as the depth of the ocean floor, which is also 20 feet below the surface. Therefore, the distance from the captain to the top of the water is the same as the distance from the diver to the ocean floor.

Related Post