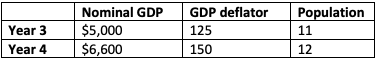
Explanation: (5,000 ÷ 125) x 100 = 4,000
Explanation: (6,600 ÷ 150) x 100 = 4,400
Explanation: (4,000 ÷ 11) x 100 = 364
Explanation: (4,400 ÷ 12) x 100 = 367
Explanation:
($100 x 1) + ($10 x 8) + ($5 x 4) = $200
Explanation:
($100 x 1) + ($10 x 10) + ($5 x 5) = $225
Explanation:
Nominal GDP = (Quantity of Apples * Price of Apples) + (Quantity of Oranges * Price of Oranges)
= (4,000 * $0.30) + (3,000 * $0.50)
= $1,200 + $1,500
= $2,700
Real GDP (from the previous question) = $2,700
GDP Deflator = (Nominal GDP / Real GDP) * 100
= ($2,700 / $2,000) * 100
= 135
Advertisement
Explanation:
Nominal GDP in Year 1 = Quantity * Price = 20,000 * $500 = $10,000,000
Nominal GDP in Year 2 = Quantity * Price = 25,000 * $450 = $11,250,000
Nominal GDP Growth Rate = [(Nominal GDP in Year 2 - Nominal GDP in Year 1) / Nominal GDP in Year 1] * 100%
= [(11,250,000 - 10,000,000) / 10,000,000] * 100%
= (1,250,000 / 10,000,000) * 100%
= 12.5%
Explanation:
To calculate the real GDP growth rate, we need to adjust for inflation by using the GDP deflator.
Real GDP in Year 1 = Nominal GDP in Year 1 / GDP Deflator in Year 1 = $500 billion / 110 = $4.545 billion
Real GDP in Year 2 = Nominal GDP in Year 2 / GDP Deflator in Year 2 = $550 billion / 120 = $4.583 billion
Real GDP Growth Rate = [(Real GDP in Year 2 - Real GDP in Year 1) / Real GDP in Year 1] * 100%
= [(4.583 - 4.545) / 4.545] * 100%
= (0.038 / 4.545) * 100%
≈ 0.84%
Therefore, the closest answer is 8%.
Explanation:
Nominal GDP increased more than real GDP because the difference between nominal and real GDP was larger in Year 2 compared to Year 1.
Explanation:
Prices went up during the year because nominal GDP, which includes the effect of price changes, increased more than real GDP, which adjusts for inflation.