GED 数学推理 4
If each letter can only be used once, how many alternative passcodes can be created using the letters A, B, C, and D?
Because each letter can only be used once and there are a total of four letters, the passcode's initial character can only be occupied by four different letters. After the first letter has been used, there are three letters that can fill the second slot, two letters for the third spot, and only one letter for the final spot. The total number of possibilities is found by multiplying these values:
4∗3∗2∗1=24
The total number of alternative arrangements of n separate elements may also be calculated using the formula:
n!=n(n−1)(n−2)…∗1
4!=4∗3∗2∗1
4!=24
Arman travels at a speed of 60 kilometers per hour from city A to city B. How many tanks of fuel will he need to drive five hours to see his family in city B if he uses 2 gallons of petrol every 50 miles? (1.6 kilometers = 1 mile)
Calculate the total distance Arman will go in 5 hours in miles. Then, using the gallons of gasoline to miles traveled ratio, figure out how many gallons of fuel he'll need for the number of miles he'll be traveling. Remember that the product of the rate of speed and the time traveled equals distance:
Distance =Rate×Time
To calculate the total distance traveled, substitute the following values:
D=60km/h∗5h
D=300 km
Arman will go 300 kilometers in five hours. To convert kilometers to miles, use the following formula:
300 km(1 mi/1.6 km)=187.5 mi
Use the ratio of gallons of fuel to miles driven to put up a proportion to solve for the total quantity of fuel necessary now that you know the total number of miles traveled:
2 gallons/50 mi = x gallons/187.5 mi
50x=187.5∗2
50x=375
x=7.5 gallons
Kate was three times Jack's age five years ago. What is Kate's age currently, if Jack is ten years old?
To keep everything structured, make a table that compares the scenario five years ago to the situation today.
Jack is now ten years old, which means he was five years old five years ago.
Kate was three times Jack's age five years ago, thus Kate must have been 15 at the time.
To compute Kate's current age, add 5 years to her age five years ago. Kate's age is now 15 + 5 = 20.
3x(2+5y) is identical to which of the following expressions?
Start by taking note of the format in which the response options are written. Start by distributing the 3x across the parenthesis because no response choice contains a parenthetical statement. Multiply each of the items inside the parenthesis by three and merge any terms that are similar:
3x(2+5y)
=3x(2)+3x(5y)
=6x+15xy
What is |ab| if a=4 and b=3?
Remember that the vertical lines that surround an equation denote absolute value. A quantity's absolute value is its positive distance from zero. For example, the absolute value of 3 is 3. Calculate the absolute value by substituting the specified values of a and b into the expression:
|a−b|
=|(−4)−3|
Remember that subtracting a negative value from a positive value produces a negative value that is bigger than the original negative value:
=|−7|
=7
从 4 男 8 女的小组中选男的概率是多少?
The ratio of successful events to the total number of occurrences determines the chance of a successful event. A successful occurrence in this scenario is the selection of a male from the group. The total number of participants in the group equals the total number of events:
P(Male)=Number of Males/Number of People
P(Male)=4/12
P(Male)=1/3
4y(3x+2)2(3x+2) is a factor.
Any element of an expression that is shared by all of its terms can be factored out. (3x+2) is a common word in this case, and it can be factored out of both sides of the expression:
4y(3x+2)−2(3x+2)
=(3x+2)[4y−2]
The original phrase is obtained by distributing (3x+2) via [4y2], which proves the answer.
Catherine saves 20% of her take-home salary after taxes at the end of each month. Which of the following expressions represents Catherine's entire savings at the end of six months if she is taxed at a 25% rate and S indicates her monthly salary before taxes?
To figure out Catherine's savings after six months, you must first figure out her monthly savings. Her monthly savings are determined by her earnings and tax rate. Begin by calculating your after-tax income:
After tax income =S−S(0.25)
After tax income =0.75S
Of this amount, Catherine saves 20%:
0.75S∗0.20=0.15S
Calculate Catherine's six-month savings by multiplying her monthly savings by six:
0.15S∗6=0.9S
A phone company will charge $2 for the first five minutes of a call, and then 30 cents per minute after that. What would Bill's total call cost be if he makes a phone call that lasts 25 minutes?
The following equation can be used to calculate the total cost of the phone call:
TC=$2.00+$0.3x, where x is the call's duration after the first five minutes. x=20 in this case. Solve for the total cost by substituting the known values into the equation:
TC=$2.00+$0.3∗20
TC=$2.00+$6.00
TC=$8.00
Which line in the diagram has a positive slope?
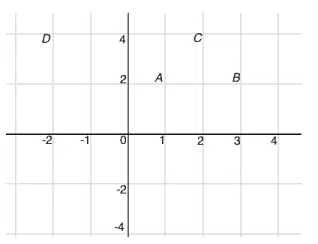
The coordinates of A are (1,2), the coordinates of C are (2,4). The slope of AC is (4-2)/( 2-1) = 2
What are the coordinates of the mirror image of point B on the x-axis in the picture?
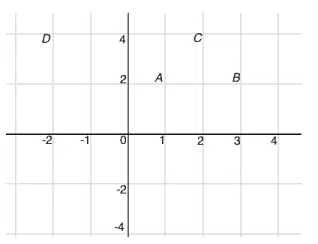
B's x-coordinate won't change if the x-axis reflects it, but it will be as far below the x-axis as it is now above the x-axis. After reflection, its coordinates will be (3, -2).
One-third of employment applicants were 25 years of age or younger. At least two-thirds of the applicants were above 50. How many of the 84 applicants fell between the ages of 25 and 50?
84 divided by 1/3 results in 84 divided by 3 and 28. There were 28 applicants that were 25 years old or younger. Two sevenths of 84 equal two and seventy-four, or 24. There were 24 applicants who were at least 50 years old. 84 – 28 – 24 = 32. There were 32 applicants for the position, ages 25 to 50.
Which of the following is both a multiple of 25 and a factor of 450?
75 is a multiple of 25 and a factor of 450.
The height of a plant, measured in inches at week t following planting, is given by the formula h - 15 = 3.2t. What is the rate of growth for the plant's height?
The height of a shrub in inches after t weeks is given by h = 15 + 3.2t. The height at planting is 15 + 3.2 0 = 15 inches. The height is 15 + 3.2 * 1 = 18.2 inches after one week. The rate of growth in height is 18.2 - 15 inches, or 3.2 inches per year.
The height of a plant t weeks after planting, measured in inches, is given by the formula h -15 = 3.2t. How tall was the shrub at its beginning?
Initial denotes the start. T is 0 at the beginning. The height of a shrub in inches after t weeks is given by h = 15 + 3.2t. The height at planting is 15 + 3.2 0 = 15 inches.
The height of a plant t weeks after planting, measured in inches, is given by the formula h -15 = 3.2t. When did the 31-inch height occur?
The height of a shrub in inches after t weeks is given by h = 15 + 3.2t. In the case where the height is 31 inches, 31 = 15 + 3.2t 3.2t = 16 t = 16÷3.2 t = 5 After 5 weeks, the height is 31 inches.
A graph showing the temperature in a refrigerator at time T (in degrees Fahrenheit) afternoon. What is the formula for this graph's symmetry axis?
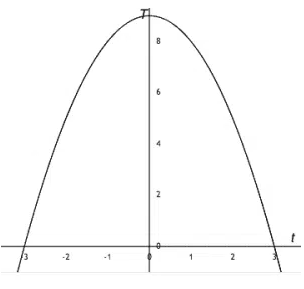
It is evident from the diagram that the T-axis serves as this graphic's mirror line or axis of symmetry. The T-axis has the equation t = 0. This graph's axis of symmetry has the equation t = 0.
A graph showing the temperature in a refrigerator at time t (after midday) in degrees Fahrenheit. Which of the following equations is the one to use to get the times when the temperature is 8^o F?
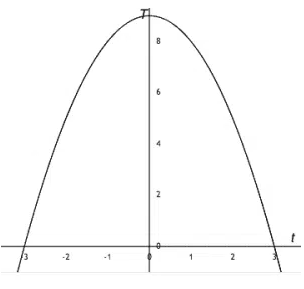
9 + t^2 = 8 is the only possible solution among the choices because it is apparent from the graph that it is not linear.
What would happen to the surface area if the sphere's radius were to double?
The smaller sphere's surface area is 12.56 m2 if r = 1. The surface area increases to 50.26 when the radius is doubled. The factor is 12.56 times 50.26. = 4. The surface area is enlarged by a factor of 4.
The sphere's volume, V, is calculated as 4(pi)r3 / 3. Which of the following represents the volume with the closest approximation when the radius is 11 inches?
In the formula, 11 must be used in place of r. The calculation is V = 4/3 x (pi=3.14) x 113 = 5572.45