FREE SATs Math Questions and Answers
Is there a function in this relation?
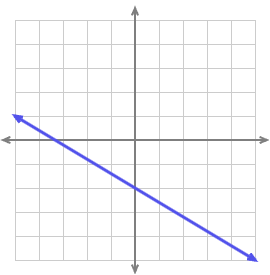
The connection is a function since it is impossible to create a vertical line that crosses the graph more than once.
Is there a function in this relation?
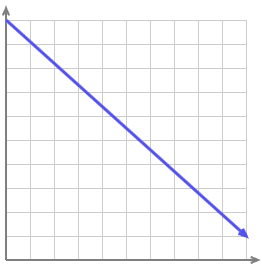
Every x-value has exactly one y-value linked with it if drawing a vertical line that crosses the graph more than once is not possible. The relation is therefore a function.
What is the effect on the graph of f(x) of the transformation f(x)→f(4x)?
The form of the transformation f(x)→f(4x) is f(x)→f(ax), where a=4. Since 4>1, it causes the f(x) graph to shrink horizontally.
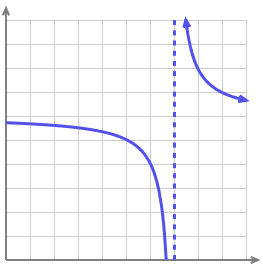
Is there a function in this relation?
Which quadrant, in standard position, contains the terminal side of a 315° angle?
The rotation is 315° counterclockwise because the angle measure is positive.
Turn the ray 315 degrees counter-clockwise such that the terminal side is in Quadrant IV.
Which quadrant, in standard position, contains the terminal side of a 135° angle?
The rotation is 135° counterclockwise because the angle measure is positive.
Turn the ray 135 degrees counter-clockwise such that the terminal side is in Quadrant II.
Which quadrant, in standard position, corresponds to the terminal side of a 225° angle?
The rotation is 225° counterclockwise because the angle measure is positive.
Turn the ray 225 degrees counter-clockwise such that the terminal side is in Quadrant III.
Advertisement
Which quadrant, in standard position, contains the terminal side of a 45° angle?
The rotation is 45° counterclockwise because the angle measure is positive.
Turn the ray 45 degrees counterclockwise so that the terminal side is in Quadrant I.
The terminal side of a 690° angle in standard position lies in which quadrant?
Since the angle measure is positive, 690° counterclockwise rotation is achieved.
The terminal side of a 480° angle in standard position lies in which quadrant?
The rotation is 480° counterclockwise because the angle measure is positive.
To begin, visualize a ray along the positive x-axis and rotate it 360° anticlockwise. Since 360° is a complete revolution, the ray is returned to its original position at the positive x-axis after this rotation.
Proceed to rotate the ray in an anticlockwise direction by another 120°, positioning the terminal side in Quadrant II.