FREE Numerical Reasoning Aptitude Questions and Answers
Which region's project cost the least money?
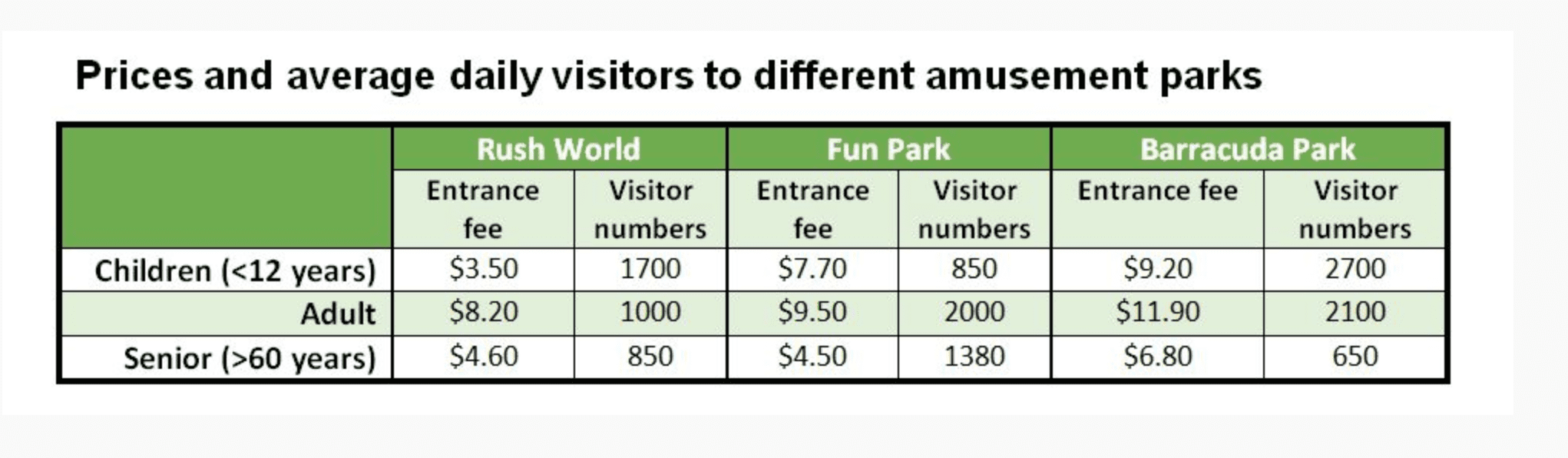
"Explanation:
Finding out how to calculate a project's cost is the first step in addressing this challenge. Inferring from the data in the table, we can say that each project's cost is solely determined by the wages that were paid during the course of the project. There are two types of salaries for any project: management and laborers. The total of the managers' and employees' pay divided by the number of months is the cost of a project. Project for the North Region: Total salary paid each month were (2 + 6 = 8) million. The project cost $192 million (8 million times 24 months). Project for the South Region: Total salary paid each month were (3.4 m + 12.3 m) = 15.7 m. The project cost $518.1 million ($15.7 million x 33 months). Project for the East Region: Total salary paid each month were (1.5 + 4.5) = 6 million. The project cost $6 million multiplied by 19 months, or $114 million. Project for the West Region: Total salary paid each month were (1 + 2.5) = 3.5 million. The project cost was $91 million ($3.5 million x 26 months). Project for the Central Region: Total salary paid each month were (6 + 9.6 m) = 15.6 m. The project cost $436.8 million ($15.7 million x 28 months). With a cost of $91 million, West Region had the lowest price."
What is the expected daily income on a Saturday if the management at Barracuda Park increased the entrance price for kids and elders by 20%?
"Explanation:
To address this question, we must calculate the new entrance prices for Barracuda Park following the price hike. The answer to the question determines a 20% increase in the entrance cost for youngsters. The new children's entrance cost will therefore be $9.20 multiplied by 100% to equal $11.04. Given that 2700 kids are likely to visit on Saturday, this category will earn $29,808 (i.e., $11.04 x 2700 kid visitors). Entrance fees for adults remain the same, hence this category's revenue will be calculated as follows: $11.90 x 2100 adult visitors = $24,990. The entrance charge rises by 20% for seniors. The revised entrance price for seniors will therefore be: $6.80 x 120% = $8.16. According to the data, there are 650 seniors who visit on average every day. This means that on Saturday, $8.16 multiplied by 650 senior visitors equals $5,304 in revenue. We must now add the anticipated earnings for the three categories to get at the solution: $29,808 + $24,990 + $5,403 = $60,102."
On a typical Monday through Thursday, roughly how many more or less persons in their 60s or under attend Rush World than Fun Park?
"Explanation:
Finding the total number of guests under the age of 60 that visited Rush World and Fun Park over the course of four weekdays can help us answer this question. There are typically 2700 visitors overall per day at Rush World, consisting of 1000 adults and 1700 kids. This amount would be 2700 x 4 = 10,800 spread over 4 days. The average daily attendance at Fun World is 2000 + 850, or 2850, which translates to 11,400 over the course of four days. As a result, Rush World receives 600 fewer visitors (11,400 – 10,800)."
The Big Cow dairy and the Cream Delight dairy have a cow-to-cow ratio of 4:5. How much milk is produced daily at each of these two dairies?
"Explanation:
You must be familiar with ratios and comprehend their meaning in order to answer this question. To calculate the ratio of milk produced by Big Cow and Cream Delight dairies, multiply the milk produced by the cow ratio. The daily milk output at Big Cow is 4.5 liters, and there are 4 cows per ratio, giving us a milk production ratio of 18 (4.5 liters 4 ratio of cows). Similar to this, Cream Delight dairy produces 4 liters of milk every day from a ratio of 5 cows. 4 litres divided by 5 cows is 20, so. Therefore, the milk production ratio between the two dairies is 18:20. By multiplying both sides of the ratio by 2, this number can be proportionally decreased to 9:10."
What is the difference in the number of cashiers in 2010 and 2007 if there were 550 workers in 2010 compared to 450 in 2007?
"Explanation:
Find the total number of cashiers in both 2007 and 2010, then subtract one from the other to arrive at the answer. As a result, the graph reveals that there were 450 employees in 2007 and that 40% of them were cashiers: 40% x 450 employees = (40/100) x 450 = 180 cashiers. Out of 550 employees, 18% were cashiers in 2010. Therefore, there are 99 cashiers, or 18% of the 550 workers, or (18/100) x 550. There are 81 cashiers between the two numbers, 180 minus 99."
Following the merger of the Big Cow and Best Fresh dairies, a block of cheese is now produced using 0.4 litres of milk on average. The Best Fresh dairy had a 2:5 advantage in terms of the number of cows in each dairy. How many cheese blocks can a typical cow create now?
"Explanation:
To find the solution to this, multiply the new combined average daily milk production per cow for the two dairies by 0.4 liters, the quantity of milk needed to make one block of cheese. The average daily milk output per cow for each dairy must be multiplied by the number of cows in the dairy, and the two values must be added to determine the new average daily milk production. According to the table, Big Cow dairy's cows produce 4.5 litres of milk every day. According to the ratio in the question, 2 / (2+5) or 2/7 of the merged new dairy's cows are in the Big Cow dairy. Therefore, 4.5 liters times 2/7 equals 9/7 of the average daily milk production at Big Cow dairy. The table indicates that Best Fresh dairy produces 3.8 litres of milk per cow each day. According to the ratio in the question, 5 / (2+5) or 5/7 of the merged new dairy's cows are in Best Fresh dairy. Therefore, 3.8 liters multiplied by 5/7 becomes 19/7, which is the percentage of the dairy's daily average milk production. Therefore, for the combined dairies, the new average daily milk output per cow is 9 + 19 + 4 = 28 + 4 = 4 litres per cow. 4 litres of milk can be used to make 10 cheese blocks, or 4 litres of milk divided by 0.4. As a result, each cow at the two dairies produces enough milk for 10 blocks of cheese."
Which region would have paid a worker the greatest income if all workers were paid equally?
"Explanation:
To address this question, we must first determine the average wage for a worker in each region. To calculate a worker's wage, divide the total worker salaries in the region by the number of workers. The North Region has 165 employees and paid a total of 6 million in wages to its workers. As a result, the wage per employee was $6 million / 165, or $36,400. With 97 employees, the South Region paid out $12.3 million in total wages to its workforce. As a result, the average wage per employee was $127,000 ($12.3 million / 97). There were 123 employees in the East Region, and a total of $4.5 million was paid in wages. The wage per employee is therefore $4.5 million / 123, or $25,000 per employee. With 118 employees, the Central Region paid $9.6 million in total wages to its workforce. The wage per employee is therefore $9.6 million / 123 = $81,400. The South Region paid the highest worker pay, at almost $127,000, according to the aforementioned statistics. If you look at the data and see that South Region pays the highest overall compensation for workers but has the fewest workers, you can determine that South Region must pay the highest worker salaries without having to calculate the number."
The amount of milk needed to make a block of cheese increased by 0.35 litres while the average daily milk production per cow at the Milk Lake dairy declined by 0.9 litres. How much money will the dairy now make per cow in cheese sales each day?
"Explanation:
By figuring out how many blocks of cheese an average cow produces and deducting the daily cost for cow maintenance, we can determine how much the Milk Lake dairy made from an average cow each day. According to the table, the new average daily milk production per cow at the Milk Lake dairy has now decreased to 4.9 - 0.9 litres = 4 litres. The new milk requirement for making a block of cheese has increased to 0.65 + 0.35 = 1 litre. As a result, the Milk Lake dairy now produces 4 blocks of cheese every day from an average cow (4 litres of milk from an average cow / 1 litre of milk per block of cheese). Since Milk Lake Dairy charges $4 per block of cheese, the daily sales revenue per cow works out to $16 per cow ($4 x 4 blocks of cheese per cow). A cow requires $10 in maintenance each day. $16 minus $10 equals $6 in profit per cow."
Which dairy generates the most cheese blocks per average cow?
"Explanation:
Calculating the number of cheese blocks that can be produced from an average cow's daily milk requires dividing the amount of milk produced by the number of cheese blocks. The table shows that each block of cheese requires 0.5 liters of milk, whereas an average cow at Bog Cow dairy produces 4.5 liters. As a result, a typical cow at this dairy makes 9 blocks of cheese (4.5 litres / 0.5 litres). Each block of cheese at Best Fresh uses 0.45 liters of milk, which is produced by an average cow in the dairy. As a result, each average cow at this dairy produces 8.45 blocks of cheese (3.8 litres / 0.45 litres). Each block of cheese at Milk Lake Dairy requires 0.65 liters of milk, which is produced by an average cow in 4.9 liters. As a result, a typical cow at this dairy makes 7.5 blocks of cheese (4.9 litres / 0.65 litres). Each block of cheese at the Cream Delight dairy requires 0.5 liters of milk, which is produced on average by one cow. A typical cow at this dairy so yields 4 litres / 0.5 litres = 8 blocks of cheese. This indicates that Big Cow dairy generates the most cheese blocks, 9 each day."
With four Australian dollars, how many Jordanian dinars can I purchase?
"Explanation:
Your proficiency with ratios will be evaluated by this question. The graph shows that 1 Jordanian dinar is equivalent to 0.8 Australian dollars. This indicates that there is a 1:1.8 ratio between the Australian dollar and the Jordanian dinar. The ratio of X:4 must be the same as the fundamental ratio between the two currencies if X is the number of Jordanian dinars I can purchase with 4 Australian dollars (i.e. 1:0.8). When dealing with ratios, we have the option of multiplying both sides by any number besides zero. The ratio's two sides must, however, be multiplied by the same number. If 0.8 x 5 is 4 Australian dollars, then the other side of the fundamental ratio must also be multiplied by 5. Thus, 1 Jordanian dinar multiplied by 5 equals 5 Jordanian dinars. Consequently, X equals 5 Jordanian dinars."
A "good" employee is one who has had four consecutive periods with no decline in revenues. How many 'good' workers exist?
"Explanation:
By implication, the question defines a ""good"" employee as one whose sales were at least equal to or higher than those in the prior quarter. Adam's sales decreased from Q1 to Q2, Chris's decreased from Q3 to Q4, and Daisy's decreased from Q2 to Q3, as seen by the graph. Tom, however, was able to boost his sales at every turn, and despite Brenda's Q2 and Q3 sales being equal, her sales never fell below those of the prior quarter. The right response is 2, as these final two meet the requirements for a ""good"" employee."